This Drawing Would Be A Step In Finding Which Point Of Concurrency In A Triangle
The point where the two altitudes intersect is the orthocenter of the triangle.
This drawing would be a step in finding which point of concurrency in a triangle. The line segment that is both perpendicular to a side of a triangle and passes through its midpoint is the. Points of concurrency concurrent lines are three or more lines that intersect at the same point. Lectures by walter lewin.
Asked 12h this drawing would be a step in finding which point of concurrency in a triangle. G is the incenter. 8 02x lect 16 electromagnetic induction faraday s law lenz law super demo duration.
Point q represents which point of concurrency. Incenter is the point of concurrency for. A point of concurrency is the point where three or more line segments or rays intersect.
Which point of concurrency is always located outside of an obtuse triangle. Point p represents which point of concurrency. In a triangle a segment connecting the midpoints of two sides of the triangle is called a.
This will help convince you that all three altitudes do in fact intersect at a single point. Let us discuss the above four points of concurrency in a triangle in detail. A segment whose endpoints are the midpoint of one side of a triangle and the opposite vertex.
M is the point of concurrency of lines m w y and x. Where must the centroid always be located regardless of acute right or obtuse. W x y the four centers of a triangle in a triangle the following sets of lines are concurrent.
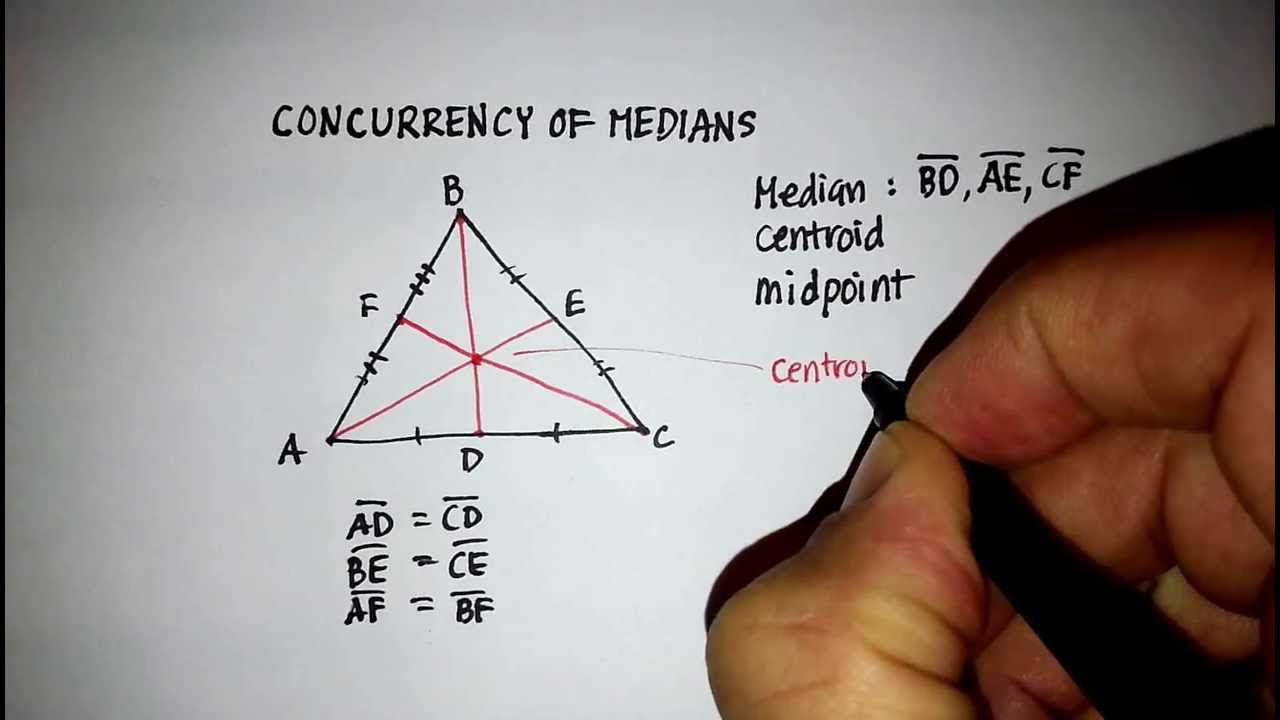
So if you constructed this three medians of each side connecting the vertex to the midpoint then you d be constructing the centroid which is also the center of gravity or the center of mass for a given triangle. Circumcenter is the point of concurrency for. You may need to extend the altitude lines so they intersect if the orthocenter is outside the triangle optional step 11.
Orthocenter is the. The point of concurrency of the medians of a triangle is called the centroid of the triangle and is usually denoted by g. Points of concurrency incenter circumcenter centroid.
So if you took your triangle and constructed your three altitudes you d be constructing a point of concurrency known as the orthocenter the last type is a median.